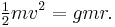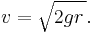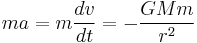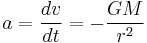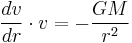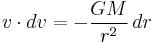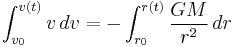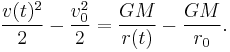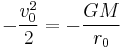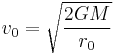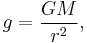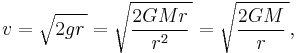Escape velocity

In physics, escape velocity is the speed at which the kinetic energy plus the gravitational potential energy of an object is zero. It is commonly described as the speed needed to "break free" from a gravitational field. The term escape velocity is actually a misnomer, as the concept refers to a scalar speed which is independent of direction.
In practice the escape velocity sets the bar for any rocket aiming to bring a satellite beyond earth orbit. It gives a minimum delta-v budget for rockets when no benefit can be obtained from the speeds of other bodies, for example on interplanetary missions where a gravitational slingshot may be applied.
When escape velocity is calculated by the gravitational potential energy (Ug) equation
atmospheric friction or air drag is neglected.
Contents |
Overview
A barycentric velocity is a velocity of one body relative to the center of mass of a system of bodies. A relative velocity is the velocity of one body with respect to another. Relative escape velocities exist only in systems with two bodies. For systems of two bodies the term "escape velocity" is ambiguous, but it is usually intended to mean the barycentric escape velocity of the less massive body. In gravitational fields "escape velocity" refers to the escape velocity of zero mass test particles relative to the barycenter of the masses generating the field.
The phenomenon of escape velocity is a consequence of conservation of energy. For an object with a given total energy, which is moving subject to conservative forces (such as a static gravity field) it is only possible for the object to reach combinations of places and speeds which have that total energy; and places which have a higher potential energy than this cannot be reached at all.
For a given gravitational potential energy at a given position, the escape velocity is the minimum speed an object without propulsion needs, to be able to "escape" from the gravity (i.e. so that gravity will never manage to pull it back). For the sake of simplicity, unless stated otherwise, we will assume that the scenario we are dealing with is that an object is attempting to escape from a uniform spherical planet by moving straight away (along a radial line away from the center of the planet), and that the only significant force acting on the moving object is the planet's gravity.
Escape velocity is actually a speed (not a velocity) because it does not specify a direction: no matter what the direction of travel is, the object can escape the gravitational field (though its path may intersect the planet). The simplest way of deriving the formula for escape velocity is to use conservation of energy. Imagine that a spaceship of mass m is at a distance r from the center of mass of the planet, whose mass is M. Its initial speed is equal to its escape velocity, 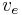 . At its final state, it will be an infinite distance away from the planet, and its speed will be negligibly small and assumed to be 0. Kinetic energy K and gravitational potential energy Ug are the only types of energy that we will deal with, so by the conservation of 'energy',
. At its final state, it will be an infinite distance away from the planet, and its speed will be negligibly small and assumed to be 0. Kinetic energy K and gravitational potential energy Ug are the only types of energy that we will deal with, so by the conservation of 'energy',
Kƒ = 0 because final velocity is zero, and Ugƒ = 0 because its final distance is infinity, so
Defined a little more formally, "escape velocity" is the initial speed required to go from an initial point in a gravitational potential field to infinity with a residual velocity of zero, with all speeds and velocities measured with respect to the field. Additionally, the escape velocity at a point in space is equal to the speed that an object would have if it started at rest from an infinite distance and was pulled by gravity to that point. In common usage, the initial point is on the surface of a planet or moon. On the surface of the Earth, the escape velocity is about 11.2 kilometers per second (~6.96 mi/s), which is approximately 34 times the speed of sound (Mach 34) and at least 10 times the speed of a rifle bullet. However, at 9,000 km altitude in "space", it is slightly less than 7.1 km/s.
The escape velocity relative to the surface of a rotating body depends on direction in which the escaping body travels. For example, as the Earth's rotational velocity is 465 m/s at the equator, a rocket launched tangentially from the Earth's equator to the east requires an initial velocity of about 10.735 km/s relative to Earth to escape whereas a rocket launched tangentially from the Earth's equator to the west requires an initial velocity of about 11.665 km/s relative to Earth. The surface velocity decreases with the cosine of the geographic latitude, so space launch facilities are often located as close to the equator as feasible, e.g. the American Cape Canaveral (latitude 28°28' N) and the French Guiana Space Centre (latitude 5°14' N).
The barycentric escape velocity is independent of the mass of the escaping object. It does not matter if the mass is 1 kg or 1,000 kg, what differs is the amount of energy required. For an object of mass  the energy required to escape the Earth's gravitational field is GMm / r, a function of the object's mass (where r is the radius of the Earth, G is the gravitational constant, and M is the mass of the Earth).
the energy required to escape the Earth's gravitational field is GMm / r, a function of the object's mass (where r is the radius of the Earth, G is the gravitational constant, and M is the mass of the Earth).
For a mass equal to a Saturn V rocket, the escape velocity relative to the launch pad is 8 nanometers per year faster than the escape velocity relative to the mutual center of mass. When the mass reaches the Andromeda galaxy the earth will have recoiled a third of a mile.
Misconception
Planetary or lunar escape velocity is sometimes misunderstood to be the speed a powered vehicle (such as a rocket) must reach to leave orbit; however, this is not the case, as the quoted number is typically the escape velocity at the body's surface, and vehicles need never achieve that speed. This barycentric escape velocity is the speed required for an object to leave the planet if the object is simply projected from the surface of the planet and then left without any more kinetic energy input: in practice the vehicle's propulsion system will continue to provide energy after it has left the surface.
In fact a vehicle can leave the Earth's gravity at any speed. At higher altitudes, the local escape velocity is lower. But at the instant the propulsion stops, the vehicle can only escape if its speed is greater than or equal to the local escape velocity at that position. As is obvious from the equation, at sufficiently high altitudes this speed approaches 0 as r becomes large.
Orbit
If an object attains escape velocity, but is not directed straight away from the planet, then it will follow a curved path. Although this path does not form a closed shape, it is still considered an orbit. Assuming that gravity is the only significant force in the system, this object's speed at any point in the orbit will be equal to the escape velocity at that point (due to the conservation of energy, its total energy must always be 0, which implies that it always has escape velocity; see the derivation above). The shape of the orbit will be a parabola whose focus is located at the center of mass of the planet. An actual escape requires of course that the orbit not intersect the planet nor its atmosphere, since this would cause the object to crash. When moving away from the source, this path is called an escape orbit. Escape orbits are called known as C3 = 0 orbits (where C3 = - μ/a, and a is the semi-major axis).
In reality there are many gravitating bodies in space, so that, for instance, a rocket that travels at escape velocity from Earth will not escape to an infinite distance away because it needs an even higher speed to escape the Sun's gravity. In other words, near the Earth, the rocket's orbit will appear parabolic, but eventually its orbit will become an ellipse around the Sun, except when it is perturbed by the Earth whose orbit it must still intersect.
List of escape velocities
| Location | with respect to | Ve[2] | Location | with respect to | Ve[2] | |
|---|---|---|---|---|---|---|
| on the Sun, | the Sun's gravity: | 617.5 km/s | ||||
| on Mercury, | Mercury's gravity: | 4.3 km/s | at Mercury, | the Sun's gravity: | 67.7 km/s | |
| on Venus, | Venus' gravity: | 10.3 km/s | at Venus, | the Sun's gravity: | 49.5 km/s | |
| on Earth, | the Earth's gravity: | 11.2 km/s | at the Earth/Moon, | the Sun's gravity: | 42.1 km/s | |
| on the Moon, | the Moon's gravity: | 2.4 km/s | at the Moon, | the Earth's gravity: | 1.4 km/s | |
| on Mars, | Mars' gravity: | 5.0 km/s | at Mars, | the Sun's gravity: | 34.1 km/s | |
| on Jupiter, | Jupiter's gravity: | 59.5 km/s | at Jupiter, | the Sun's gravity: | 18.5 km/s | |
| on Saturn, | Saturn's gravity: | 35.6 km/s | at Saturn, | the Sun's gravity: | 13.6 km/s | |
| on Uranus, | Uranus' gravity: | 21.2 km/s | at Uranus, | the Sun's gravity: | 9.6 km/s | |
| on Neptune, | Neptune's gravity: | 23.6 km/s | at Neptune, | the Sun's gravity: | 7.7 km/s | |
| in the solar system, | the Milky Way's gravity: | ≥ 525 km/s [3] | ||||
| on the event horizon, | the black hole's gravity: | >299,792 km/s |
Because of the atmosphere it is not useful and hardly possible to give an object near the surface of the Earth a speed of 11.2 km/s, as these speeds are too far in the hypersonic regime for most practical propulsion systems and would cause most objects to burn up due to atmospheric compression or be torn apart by atmospheric friction. For an actual escape orbit a spacecraft is first placed in low Earth orbit and then accelerated to the escape velocity at that altitude, which is a little less — about 10.9 km/s. The required acceleration, however, is generally far less because from a low Earth orbit the spacecraft already has a speed of approximately 8 km/s.
Calculating an escape velocity
To expand upon the derivation given in the Overview,
where 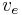 is the barycentric escape velocity, G is the gravitational constant, M is the mass of the body being escaped from, r is the distance between the center of the body and the point at which escape velocity is being calculated, g is the gravitational acceleration at that distance, and μ is the standard gravitational parameter.[4]
is the barycentric escape velocity, G is the gravitational constant, M is the mass of the body being escaped from, r is the distance between the center of the body and the point at which escape velocity is being calculated, g is the gravitational acceleration at that distance, and μ is the standard gravitational parameter.[4]
The escape velocity at a given height is 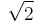 times the speed in a circular orbit at the same height, (compare this with equation (14) in circular motion). This corresponds to the fact that the potential energy with respect to infinity of an object in such an orbit is minus two times its kinetic energy, while to escape the sum of potential and kinetic energy needs to be at least zero. The velocity corresponding to the circular orbit is sometimes called the first cosmic velocity, whereas in this context the escape velocity is referred to as the second cosmic velocity"[5]
times the speed in a circular orbit at the same height, (compare this with equation (14) in circular motion). This corresponds to the fact that the potential energy with respect to infinity of an object in such an orbit is minus two times its kinetic energy, while to escape the sum of potential and kinetic energy needs to be at least zero. The velocity corresponding to the circular orbit is sometimes called the first cosmic velocity, whereas in this context the escape velocity is referred to as the second cosmic velocity"[5]
For a body with a spherically-symmetric distribution of mass, the barycentric escape velocity 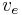 from the surface (in m/s) is approximately 2.364×10−5 m1.5kg−0.5s−1 times the radius r (in meters) times the square root of the average density ρ (in kg/m³), or:
from the surface (in m/s) is approximately 2.364×10−5 m1.5kg−0.5s−1 times the radius r (in meters) times the square root of the average density ρ (in kg/m³), or:
Deriving escape velocity using calculus
These derivations use calculus, Newton's laws of motion and Newton's law of universal gravitation.
Derivation using only g and r
the Earth's escape speed can be derived from "g," the barycentric acceleration due to gravity at the Earth's surface. It is not necessary to know the gravitational constant G or the mass M of the Earth. Let
- r = the Earth's radius, and
- g = the barycentric acceleration of gravity at the Earth's surface.
Above the Earth's surface, the acceleration of gravity is governed by Newton's inverse-square law of universal gravitation. Accordingly, the acceleration of gravity at height s above the center of the Earth (where s > r ) is g (r / s)2. The weight of an object of mass m at the surface is g m, and its weight at height s above the center of the Earth is gm (r / s)2. Consequently the energy needed to lift an object of mass m from height s above the Earth's center to height s + ds (where ds is an infinitesimal increment of s) is gm (r / s)2 ds. Since this decreases sufficiently fast as s increases, the total energy needed to lift the object to infinite height does not diverge to infinity, but converges to a finite amount. That amount is the integral of the expression above:
That is how much kinetic energy the object of mass m needs in order to escape. The kinetic energy of an object of mass m moving at speed v is (1/2)mv2. Thus we need
The factor m cancels out, and solving for v we get
If we take the radius of the Earth to be r = 6400 kilometers and the acceleration of gravity at the surface to be g = 9.8 m/s2, we get
This is just a bit over 11 kilometers per second, or a bit under 7 miles per second, as Isaac Newton calculated.
Derivation using G and M
Let G be the gravitational constant and let M be the mass of the earth and 'm' be the mass of other body to be escaped.
By applying the chain rule, we get:
Because 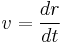
Since we want escape velocity
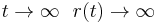 and
and 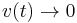
v0 is the escape velocity and r0 is the radius of the planet. Note that the above derivation relies on the equivalence of inertial mass and gravitational mass.
The derivations are consistent
The barycentric gravitational acceleration can be obtained from the gravitational constant G and the mass of Earth M:
where r is the radius of Earth. Thus
so the two derivations given above are consistent.
Multiple sources
The escape velocity from a position in a field with multiple sources at rest with respect to each other is derived from the total potential energy per kg at that position, relative to infinity. The potential energies for all sources can simply be added. For the escape velocity this results in the square root of the sum of the squares of the escape velocities of all sources separately.
For example, at the Earth's surface the escape velocity for the combination Earth and Sun would be  , if the Earth were stationary with respect to the sun. However. giving an object this speed in the direction of the Earth's orbital velocity would not be enough. because the total energy of the object is not conserved: it loses more kinetic energy (with respect to the Sun) than the gain of potential energy. With the difference the Earth recovers some of the kinetic energy it lost when the object was launched. Or put differently: the object has to do more work to overcome the Earth's gravity than when the Earth were stationary, because for a given distance covered the increase of the distance to the Earth is less, and hence the reduction of the force is less.
, if the Earth were stationary with respect to the sun. However. giving an object this speed in the direction of the Earth's orbital velocity would not be enough. because the total energy of the object is not conserved: it loses more kinetic energy (with respect to the Sun) than the gain of potential energy. With the difference the Earth recovers some of the kinetic energy it lost when the object was launched. Or put differently: the object has to do more work to overcome the Earth's gravity than when the Earth were stationary, because for a given distance covered the increase of the distance to the Earth is less, and hence the reduction of the force is less.
The minimum effective escape velocity is reduced by the orbital velocity in a different way. In the circular orbit approximation the escape velocity with respect to the Sun would be multiplied by a factor of 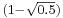 . Therefore the minimum actual combined Earth-Sun escape velocity from the Earth's surface is
. Therefore the minimum actual combined Earth-Sun escape velocity from the Earth's surface is 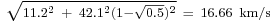 . As a result, to leave the solar system requires a speed of 16.7 km/s from the Earth's surface; in the direction of the Earth's orbital motion.
. As a result, to leave the solar system requires a speed of 16.7 km/s from the Earth's surface; in the direction of the Earth's orbital motion.
Dynamic situations
The escape velocity calculation assumes that none of the sources of gravitational potential are moving. In the case of the Earth-Moon system for example the Moon is in orbit around the Earth, and it turns out that an object that passes behind the moon can gain speed from the encounter (a gravitational assist).
Therefore there are certain launch angles and launch windows that will give the assist and this will give an object extra speed in-flight and still permit it to escape Earth's gravity even though it was launched with less than escape velocity.
Gravity well
In the hypothetical case of uniform density, the velocity that an object would achieve when dropped in a hypothetical vacuum hole from the surface of the Earth to the center of the Earth is the escape velocity divided by 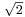 , i.e. the speed in a circular orbit at a low height. Correspondingly, the escape velocity from the center of the Earth would be
, i.e. the speed in a circular orbit at a low height. Correspondingly, the escape velocity from the center of the Earth would be 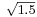 times that from the surface.
times that from the surface.
A refined calculation would take into account the fact that the Earth's mass is not uniformly distributed as the center is approached. This gives higher speeds.
See also
- Characteristic energy (C3)
- Delta-v budget – speed needed to perform manoeuvres.
- Gravitational slingshot – three-body technique for gaining energy
- Gravity well
- Two-body problem
- Black hole – An object with an escape velocity greater than the speed of light
- Oberth effect – burning fuel deep in a gravity field gives higher velocity
References
- Roger R. Bate, Donald D. Mueller, and Jerry E. White (1971). Fundamentals of astrodynamics. New York: Dover Publications. ISBN 0-486-60061-0.
- ↑ http://nssdc.gsfc.nasa.gov/nmc/spacecraftDisplay.do?id=1959-012A
- ↑ 2.0 2.1 "Solar System Data". Georgia State University. http://hyperphysics.phy-astr.gsu.edu/hbase/solar/soldata2.html. Retrieved 2007-01-21.
- ↑ [1]
- ↑ Bate, Mueller and White, p. 35
- ↑ Teodorescu, P. P. (2007). Mechanical systems, classical models. Springer, Japan. p. 580. ISBN 1-402-05441-6. http://books.google.com/books?id=k4H2AjWh9qQC&pg=PA580., Section 2.2.2, p. 580
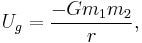

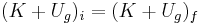
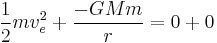
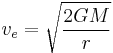

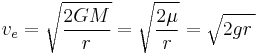
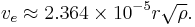
![\begin{align}
\int_r^\infty gm \left(\frac{r}{s}\right)^2 \, ds
& = gmr^2 \int_r^\infty s^{-2}\,ds
= gmr^2 \left[-s^{-1}\right]_{s:=r}^{s:=\infty} \\
& = gmr^2\left(0-(-r^{-1})\right)=gmr.
\end{align}](/I/45a05fc05991c8dd772c24310d8d7a86.png)